五維多胞體
 五維正六胞體 |
 五维正轴体, 211 |
 五维超正方体 |
 擴展五維正六胞體 |
 截半五维正轴体 |
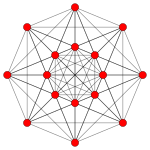 五维半立方體 121 |
在五維幾何學中,五維多胞體又稱5-多胞形,是由多個四維多胞體作為維面所構成的封閉幾何結構,每個四維胞中的三維胞(多面體)都是2個四維胞的公共胞。
這些多胞體的組成元素可分為四維胞、三維胞、面、稜和頂點,其中四維胞又稱為此幾何結構的維面;三維胞又稱為此幾何結構的維稜;二維的面又稱為此幾何結構的維峰,維面、維脊和維峰可以視為三維多面體之面、稜和頂點在五維多胞體的類比。
五維空間中至少需具有6個四維胞才能構成不退化的五維多胞體,為六胞體。而四維胞數最少的五維正多胞體亦由6個四維胞組成,稱為五維正六胞體,由6個全等的四維正五胞體組成。
定義
[编辑]五維多胞體是位於五維空間的封閉幾何結構,組成元素包括了頂點、邊、面、三維胞和四維胞,每個頂點都至少是五條邊的公共頂點;每條邊都至少是四個面的公共邊;每個面也都至少是三個多面體胞的公共面,其三維胞要是不退化的多面體;維面(或四維胞)也要是不退化的四維多胞體。
此外,也需滿足下列條件:

以五維超正方體為例:
因此五維超正方體是一種五維多胞體。
性質
[编辑]任一五維多胞體,其拓樸結構可以由其扭轉係數和貝蒂數定義[1]。
用於表徵多面體的歐拉特徵數無法十分有效地推廣到四維或更高維度,換句話說,即歐拉特徵數無法有效地區分在五維空間中的五維多胞體之不同拓撲結構,由於歐拉特徵數不足以可靠地區分更高維度的不同拓撲結構導致發現了更複雜的貝蒂數[1]。
正多胞體
[编辑]五維空間中共存在三種凸正多胞體,即五維空間只有三種凸的有限幾何結構滿足所有四維胞全等、三維胞全等、面全等、所有稜等長、所有角等角的特性。
五維空間的正多胞體在施萊夫利符號中皆可以用{p,q,r,s}表示,代表每個二維的面都是s個{p,q,r}四維胞的公共面。
三種五維凸正多胞體的施萊夫利符號分別為:
| 名稱 | 施萊夫利符號 | 考克斯特記號 | 頂點 | 邊 | 面 | 三維胞 | 四維胞 | 對稱性 (階數) |
|---|---|---|---|---|---|---|---|---|
| 五維正六胞體 | {3,3,3,3} | 6 | 15 | 20 | 15 | 6 | A5, (120) | |
| 五维超正方体 | {4,3,3,3} | 32 | 80 | 80 | 40 | 10 | BC5, (3820) | |
| 五维正轴体 | {3,3,3,4} {3,3,31,1} |
10 | 40 | 80 | 80 | 32 | BC5, (3840) 2×D5 |
均勻多胞體
[编辑]在五維幾何學中,五維均勻多胞體或五維半正多胞體是指具有點可遞的五維多胞體。[2]
其中三種五維半正多胞體的四維胞、三維胞、面、稜和頂點的數量分別為:
| 名稱 | 施萊夫利符號 | 考克斯特記號 | 頂點 | 邊 | 面 | 三維胞 | 四維胞 | 對稱性 (階數) |
|---|---|---|---|---|---|---|---|---|
| 擴展五維正六胞體 | t0,4{3,3,3,3} | 30 | 120 | 210 | 180 | 162 | 2×A5, (240) | |
| 五维半立方體 | {3,32,1} h{4,3,3,3} |
16 | 80 | 160 | 120 | 26 | D5, (1920) ½BC5 | |
| 截半五维正轴体 | t1{3,3,3,4} t1{3,3,31,1} |
40 | 240 | 400 | 240 | 42 | BC5, (3840) 2×D5 |
參考文獻
[编辑]- ^ 1.0 1.1 Richeson, D.; Euler's Gem: The Polyhedron Formula and the Birth of Topoplogy, Princeton, 2008.
- ^ Klitzing, Richard. 5D uniform polytopes (polytera). bendwavy.org.
- T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
- A. Boole Stott: Geometrical deduction of semiregular from regular polytopes and space fillings, Verhandelingen of the Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910
- H.S.M. Coxeter:
- H.S.M. Coxeter, M.S. Longuet-Higgins und J.C.P. Miller: Uniform Polyhedra, Philosophical Transactions of the Royal Society of London, Londne, 1954
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1](页面存档备份,存于互联网档案馆)
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
- Klitzing, Richard. 5D uniform polytopes (polytera). bendwavy.org.
外部連結
[编辑]- Polytopes of Various Dimensions(页面存档备份,存于互联网档案馆), Jonathan Bowers
- Uniform Polytera(页面存档备份,存于互联网档案馆), Jonathan Bowers
- Multi-dimensional Glossary(页面存档备份,存于互联网档案馆), Garrett Jones
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.
