在数学中，测度是一種將几何空間的度量（长度、面积、体积）和其他常见概念（如大小、质量和事件的概率）廣義化後產生的概念。传统的黎曼积分是在区间上进行的，為了把积分推广到更一般的集合上，人們就发展出测度的概念。一个特别重要的例子是勒贝格测度，它從 ${\displaystyle n}$ 维欧式空间 ${\displaystyle {\mathbb {R} }^{n))$ 出發，概括了傳統长度、面积和体积等等的概念。

研究測度的學問被統稱為测度论，因為指定的數值通常是非負实数，所以测度论通常會被視為实分析的一个分支，它在数学分析和概率论有重要的地位。

正式定义

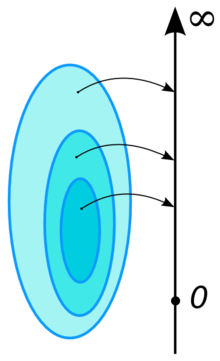
直觀上，測度是「體積」的推廣；因為空集合的「體積」當然為零，而且互相獨立的一群（可數個）物體，總「體積」當然要是所有物體「體積」直接加總（的極限）。而要定義「體積」，必須先要決定怎樣的一群子集合，是「可以測量的」，詳細請見σ-代數。

如果將 ${\displaystyle \mu }$ 的值域擴展到複數，也就是說 ${\displaystyle \mu :\Sigma \to \mathbb {C} }$ ，那 ${\displaystyle \mu }$ 會被進一步稱為複數測度。^[1]

定義的分歧

若照著上述定義，根據可数可加性，不少母集合本身的測度值會變成无穷大（如對 ${\displaystyle {\mathbb {R} }^{n))$ 本身取勒贝格测度），所以實際上不存在。但某些書籍^[2]會形式上將无穷大視為一個數，而容許測度取值為無窮大；這樣定義的書籍，會把只容許有限实数值的測度稱為（非負）有限測度。但這樣"定義"，會造成可數可加性與數列收斂的定義產生矛盾。

所以要延續體積是一種＂度量＂的這種直觀概念（也就是嚴謹的定義勒贝格测度），那就必須把σ-代數換成條件比較寬鬆的半集合環（英语：Semiring#Semiring_of_sets），然後以此為基礎去定義一個對應到＂體積＂的前測度（英语：Pre-measure）。

更進一步的，如果對測度空間 ${\displaystyle (X,\,\Sigma ,\,\mu )}$ 來說，母集合 ${\displaystyle X}$ 可表示為 ${\displaystyle \Sigma }$ 內的某可測集合序列 ${\displaystyle \{E_{n}\in \Sigma \}_{n\in \mathbb {N} ))$ 的并集：

${\displaystyle X=\bigcup _{n\in \mathbb {N} }E_{n))$

且 ${\displaystyle \mu }$ 只容許取有限值，則 ${\displaystyle \mu }$ 會被進一步的稱為（非負）σ-有限测度。

性质

单调性

测度${\displaystyle \mu \ }$的单调性： 若${\displaystyle E_{1}\ }$和${\displaystyle E_{2}\ }$为可测集，而且${\displaystyle E_{1}\subseteq E_{2))$，则${\displaystyle \mu (E_{1})\leq \mu (E_{2})}$。

可数个可测集的并集的测度

若${\displaystyle E_{1},E_{2},E_{3}\cdots }$为可测集（不必是两两不交的），则集合${\displaystyle E_{n}\ }$的并集是可测的，且有如下不等式（「次可列可加性」）：

${\displaystyle \mu (\bigcup _{i=1}^{\infty }E_{i})\leq \sum _{i=1}^{\infty }\mu (E_{i})}$

如果还满足并且对于所有的${\displaystyle n\ }$，${\displaystyle E_{n}\ }$⊆${\displaystyle E_{n+1}\ }$，则如下极限式成立：

${\displaystyle \mu \left(\bigcup _{i=1}^{\infty }E_{i}\right)=\lim _{i\to \infty }\mu (E_{i}).}$

可数个可测集的交集的测度

若${\displaystyle E_{1},E_{2},\cdots }$为可测集，并且对于所有的${\displaystyle n\ }$，${\displaystyle E_{n+1}\ }$⊆${\displaystyle E_{n}\ }$，则${\displaystyle E_{n}\ }$的交集是可测的。进一步说，如果至少一个${\displaystyle E_{n}\ }$的测度有限，则有极限：

${\displaystyle \mu (\bigcap _{i=1}^{\infty }E_{i})=\lim _{i\to \infty }\mu (E_{i})}$

如若不假设至少一个${\displaystyle E_{n}\ }$的测度有限，则上述性质一般不成立。例如对于每一个${\displaystyle n\in \mathbb {N} }$，令

${\displaystyle E_{n}=[n,\infty )\subseteq \mathbb {R} }$

这裡，全部集合都具有无限测度，但它们的交集是空集。

完备性

直觀上，因為測度的單調性，只要包含於零測集的集合，也「應該」是零測集，完備測度的定義體現了這個直觀的想法。更進一步的，任意测度可以按如下的定理擴展为完备测度：^[3]

例子

下列是一些测度的例子（顺序与重要性无关）。

• 计数测度　定义为${\displaystyle \mu (S)=S\ }$的「元素个数」。
• 一维勒贝格测度是定义在${\displaystyle \mathbb {R} }$的一个含所有区间的σ代数上的、完备的、平移不变的、满足${\displaystyle \mu ([0,1])=1\ }$的唯一测度。
• Circular angle测度是旋转不变的。
• 局部紧拓扑群上的哈尔测度是勒贝格测度的一种推广，而且也有类似的刻划。
• 恆零测度定义为${\displaystyle \mu (S)=0\ }$，对任意的${\displaystyle S\ }$。
• 每一个概率空间都有一个测度，它对全空间取值为1（于是其值全部落到单位区间[0,1]中）。这就是所谓概率测度。见概率论公理。

其它例子，包括：狄拉克测度、波莱尔测度、若尔当测度、遍历测度、欧拉测度、高斯测度、贝尔测度、拉东测度。

相关条目

• 外测度（Outer measure）
• 幾乎處處（Almost everywhere）
• 勒贝格测度（Lebesgue measure）
• 勒貝格積分
• 法圖引理(Fatou's lemma)
• 富比尼定理(Fubini's theorem)
• 可測基數

参考文献

• R. M. Dudley, 2002. Real Analysis and Probability. Cambridge University Press.
• D. H. Fremlin, 2000. Measure Theory. Torres Fremlin.
• Paul Halmos, 1950. Measure theory. Van Nostrand and Co.
• M. E. Munroe, 1953. Introduction to Measure and Integration. Addison Wesley.
• Shilov, G. E., and Gurevich, B. L., 1978. Integral, Measure, and Derivative: A Unified Approach, Richard A. Silverman, trans. Dover Publications. ISBN 0-486-63519-8. Emphasizes the Daniell integral.

外部链接

• Hazewinkel, Michiel (编), Measure, 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4 
• Tutorial: Measure Theory for Dummies（为初学者准备的测度论教学） （页面存档备份，存于互联网档案馆）