抛物线的求积

对抛物线的求积(希腊语:Τετραγωνισμὸς παραβολῆς)是古希腊数学家阿基米德的一篇几何学论文,成文于公元前三世纪。此论文源自阿基米德寄往他的朋友多西修斯的信中(其中包括有关抛物线的24个命题),最终证明了抛物线与直线之间的面积是其内切三角形的面积的 倍。
论文中陈述使用的是穷竭法。阿基米德将所求区域分割成无限个三角形,三角形的面积则形成了一个等比数列。阿基米德计算了这个等比数列的加和,然后证明了这个加和是抛物线圆缺的面积。此为古代数学穷竭法中最精妙的用法,直到17世纪积分学的发展,卡瓦列里的求积公式取代它之前,它一直是无与伦比的。[1]
理论概述
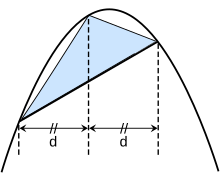
此处的弓形是指由抛物线和直线结合的区域。[2]为了求得弓形的面积,阿基米德考虑了一个内接确定的三角形,这个三角形的底是抛物线的弦,除弦上两点之外的三角形第三点在抛物线上的切线平行于弦,根据命题1(抛物线的求积),如果从第三个顶点画一条平行于轴线的线能将弦分成相等的两条线段,则抛物线弓形的面积是该内接三角形面积的4/3。
论文结构
阿基米德给出了主定理的两个证明。 第一种使用力学,阿基米德认为,当放置在适当的杠杆上的一个位置上时,线段的“重量”将能够平衡三角形的“重量”。 第二个更著名的证明是使用纯几何,特别是穷竭法。
在这二十四个命题中,前三个命题都未经欧几里得的《圆锥曲线要点》(欧几里得在圆锥曲线研究中丢的一部著作)所引用。 命题四和命题五确定了抛物线的基本性质; 命题6至17给出了主定理的机械证明; 命题18至24给出了几何证明。
几何证明
分割抛物线

证明的主要思想是将抛物线与线段之间的面积分割为无数个三角形。如右图所示,每一个三角形都以同样的方式内接于一个抛物线弓形,比如蓝色三角形被整个弓形面积所内接,绿色三角形则内接于蓝色三角形占据后剩下面积的弓形中。
三角形的面积
在第18至21个命题中,阿基米德证明了每个绿色三角形的面积是蓝色三角形面积的八分之一。 从现代数学的角度来看,这是因为绿色三角形的宽度为一半,高度的四分之一。[3]

通过扩展,每个黄色三角形的面积是绿色三角形的八分之一,每个红色三角形的面积是黄色三角形的八分之一,依此类推。 使用穷竭法,可以得出抛物线段的总面积为
这里的 T 代表大蓝色三角形的面积,第二项代表两个绿色三角形的总面积,第三项代表四个黄色三角形的总面积,依此类推。 可以很容易的得出[4]
级数求和
为了完成证明,阿基米德证明了
上面的式子是等比数列—每个连续项是前一项的四分之一。在现代数学中,该公式是等比数列的求和公式的特例。
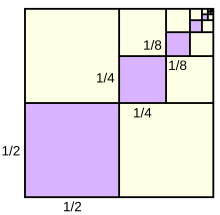
这里阿基米德使用纯粹的几何的方法求和。[5]如图所示。 此图片显示了一个单位正方形,该正方形已被分解为多个较小的正方形。 每个连续的紫色方块的面积为前一个方块的四分之一,紫色的总面积为总和。
但是,每个紫色正方形和与之对应的一组黄色正方形中的任何一个都全等,因此紫色正方形的面积覆盖了单位正方形面积的1/3。 因此,上述序列之和为4/3。[6]
其他
注解
- ^ Victor J. Katz (1995), "Ideas of Calculus in Islam and India", Mathematics Magazine 68 (3): 163–174 [165–9 & 173–4]
- ^ Weisstein, Eric W. (编). Parabolic Segment. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. [2020-07-28]. (原始内容存档于2020-03-18) (英语).
- ^ 绿色三角形在构造上具有蓝色三角形的宽度的一半。 关于高度的特征是根据抛物线的几何特性得出的,很容易使用现代的解析几何进行证明
- ^ 阿基米德"抛物线求积法"的简易情形证明. 数学之家. [2020-07-28]. (原始内容存档于2020-12-04).
- ^ 严格来说,阿基米德研究该级数的部分求和,并使用阿基米德公理认为部分求和变得无限趋近于4 / 3。 从逻辑上讲,这等效于现代数学的无穷级数求和。
- ^ 黄有度; 苏化明. 一個歷史名題的注記 (PDF). [2020-07-28]. (原始内容存档 (PDF)于2020-10-20).
延展阅读
- Ajose, Sunday and Roger Nelsen. Proof without Words: Geometric Series. Mathematics Magazine. June 1994, 67 (3): 230. JSTOR 2690617. doi:10.2307/2690617.
- Ancora, Luciano. Quadrature of the parabola with the square pyramidal number. Archimede. 2014, 66 (3) [2020-07-27]. (原始内容存档于2018-07-25).
- Bressoud, David M. A Radical Approach to Real Analysis 2nd. Mathematical Association of America. 2006. ISBN 0-88385-747-2..
- Dijksterhuis, E.J. (1987) "Archimedes", Princeton U. Press ISBN 0-691-08421-1
- Edwards Jr., C. H. The Historical Development of the Calculus 3rd. Springer. 1994. ISBN 0-387-94313-7..
- Heath, Thomas L. The Works of Archimedes 2nd. CreateSpace. 2011. ISBN 978-1-4637-4473-1.
- Simmons, George F. Calculus Gems. Mathematical Association of America. 2007. ISBN 978-0-88385-561-4..
- Stein, Sherman K. Archimedes: What Did He Do Besides Cry Eureka?. Mathematical Association of America. 1999. ISBN 0-88385-718-9.
- Stillwell, John. Mathematics and its History 2nd. Springer. 2004. ISBN 0-387-95336-1..
- Swain, Gordon and Thomas Dence. Archimedes' Quadrature of the Parabola Revisited. Mathematics Magazine. April 1998, 71 (2): 123–30. JSTOR 2691014. doi:10.2307/2691014.
- Wilson, Alistair Macintosh. The Infinite in the Finite. Oxford University Press. 1995. ISBN 0-19-853950-9..
外部链接
- Casselman, Bill. Archimedes' quadrature of the parabola. [2020-07-27]. (原始内容存档于2006-12-17). Full text, as translated by T.L. Heath.
- Xavier University Department of Mathematics and Computer Science. Archimedes of Syracuse. [2020-07-27]. (原始内容存档于2007-03-07). Text of propositions 1–3 and 20–24, with commentary.
- http://planetmath.org/ArchimedesCalculus (页面存档备份,存于互联网档案馆)
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.