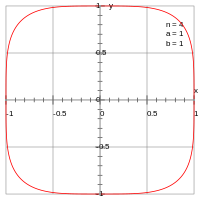
超橢圓（英語：superellipse）也稱為拉梅曲線（Lamé curve），是在笛卡儿坐标系下滿足以下方程式的點的集合：

${\displaystyle |{\frac {x}{a))|^{n}\!+|{\frac {y}{b))|^{n}\!=1}$

其中n、a及b為正數。

上述方程式的解會是一個在−a ≤ x ≤ +a及−b ≤ y ≤ +b長方形內的封閉曲線，參數a及b稱為曲線的半直徑（semi-diameters）。

n在0和1之間時，超橢圓的圖形類似一個曲線的四角星，四邊的曲線往內凹。

n為1時，超橢圓的圖形為一菱形，四個頂點為（±a, 0）及（0, ±b）。n在1和2之間時，超橢圓的圖形類似菱形，四個頂點位置相同，但四邊是往外凸的曲線，越接近頂點，曲線的曲率越大，頂點的曲率趨近無限大。

n為2時，超橢圓的圖形即為橢圓（若a = b時則為一個圓形）。當n大於2時，超橢圓的圖形看似四角有圓角（英语：Chamfer）的長方形，曲線的曲率在（±a, 0）及（0, ±b）四點為0。n為4的超橢圓也稱為方圓形。

n < 2的超橢圓也稱為次椭圆（hypoellipse），n > 2的超橢圓則稱為過椭圆（hyperellipse）。

當n ≥ 1，且a = b=1時的超橢圓是二維L^p空间下的單位圓，n即為其p-範數。

超橢圓的極點為（±a, 0）及（0, ±b），而其四個「角」為（±sa, ±sb），其中 ${\displaystyle s=2^{-{\frac {1}{n))))$。

數學性質

當n為一個非零的有理數p/q（最簡分數形式），則超橢圓為一平面代數曲線。若n為正數，其曲線次數為pq，若n為負數，其曲線次數為2pq。若a和b均為1且n為偶數，則此超橢圓為一n次的費馬曲線（英语：Fermat curve），此時超橢圓沒有奇點，但一般而言超橢圓中會有有奇點。

超橢圓的參數方程如下：

${\displaystyle \left.{\begin{aligned}x\left(\theta \right)&=\pm a\cos ^{\frac {2}{n))\theta \\y\left(\theta \right)&=\pm b\sin ^{\frac {2}{n))\theta \end{aligned))\right\}\qquad 0\leq \theta <{\frac {\pi }{2))}$

或

${\displaystyle {\begin{aligned}x\left(\theta \right)&={|\cos \theta }|^{\frac {2}{n))\times a\operatorname {sgn}(\cos \theta )\\y\left(\theta \right)&={|\sin }\theta |^{\frac {2}{n))\times b\operatorname {sgn}(\sin \theta )\end{aligned))}$

超橢圓內的面積可以用Γ函数Γ(x)來表示：

${\displaystyle \ S}$ = ${\displaystyle \Gamma (x)=4ab{\frac {\left(\Gamma \left(1+{\tfrac {1}{n))\right)\right)^{2)){\Gamma \left(1+{\tfrac {2}{n))\right))).}$

其垂足曲線較容易計算，而以下曲線的垂足曲線

${\displaystyle \left({\frac {x}{a))\right)^{n}\!+\left({\frac {y}{b))\right)^{n}\!=1}$

可以用極坐標方式來表示^[1]：

${\displaystyle (a\cos \theta )^{\tfrac {n}{n-1))+(b\sin \theta )^{\tfrac {n}{n-1))=r^{\tfrac {n}{n-1)).}$

延伸

超橢圓可以延伸為以下的形式：

${\displaystyle \left|{\frac {x}{a))\right|^{m}+\left|{\frac {y}{b))\right|^{n}=1;\qquad m,n>0.}$

或

${\displaystyle {\begin{aligned}x\left(\theta \right)&={|\cos \theta |}^{\frac {2}{m))\cdot a\operatorname {sgn}(\cos \theta )\\y\left(\theta \right)&={|\sin \theta |}^{\frac {2}{n))\cdot b\operatorname {sgn}(\sin \theta ).\end{aligned))}$

其中的${\displaystyle \theta }$不是表示角度，只是方程式的一個參數。

歷史

超橢圓在笛卡兒坐標系下的表示式是由1795年出生的法國數學家加布里埃爾·拉梅，由椭圓的方程式擴展而得。

字體設計師赫爾曼·察普夫在1952年設計的Melior（英语：Melior）字體，利用超橢圓作為字母o的外形。三十年後高德納設法選擇了介於橢圓及超橢圓之間的曲線（兩者都用样条函数近似），作為他的Computer Modern字體。

1959年時瑞典斯德哥尔摩提出了其市中心賽格爾廣場圓環的設計競賽。丹麥詩人皮亞特·海恩(1905–1996)的設計以是一個n = 2.5，a/b = 6/5的超橢圓為基礎^[2]。他的說明如下：

人是唯一一種會畫線然後將自己絆倒的動物。整個文明的推進有二個不同的取向：一種以直線及長方形為主，另一種則圓弧線為主。二種取向都有其機構上及心理上的原因。直線的事物可以放在一起，節省空間。而圓的東西很簡單，容易移動。但我們常常會陷入要在二者中選擇一個的困境，此時往往是介於二者中間的事物會更合適。隨意繪製的作品－例如以往在斯德哥尔摩出現過的圓環－無法達到這一點。它不是一個固定的形狀，也不像圓或方形有明確的定義，在美感上有所不足。超橢圓解決了這一個問題，它介於圓和長方形之間，既不是圓也不是長方形。它是一個有固定形狀、有明確定義的一個整體。

賽格爾廣場在1967年完成，而皮亞特·海恩繼續在其他的藝術品中使用超橢圓，包括牀、碟子、桌子等^[3]。皮亞特·海恩將超橢圓以長軸為軸心旋轉，形成了一個立體的超級蛋（英语：superegg），其特點是可以平面上直立，不會倒下，因此變成一個特別的玩具。

1968年在巴黎在為越戰談判時，談判者不滿意談判桌的外形，Balinski、Kieron Underwood及Holt在一封寄給紐約時報的信件中建議以超橢圓作為談判桌的外形^[2]。1968年由墨西哥城主辦奧運時，也以超橢圓為阿茲特克體育場的外形。

沃尔多·托布勒在1973年提出了托布勒超橢圓投影（英语：Tobler hyperelliptical projection）^[4]，其中的經線就是用超橢圓來表示。

美式足球球隊匹兹堡钢人的標誌是三個相連的超橢圓。

相關條目

• 星形线，n = 2/3，且a = b的超橢圓，是四尖瓣的內擺線。
  • 三尖瓣線（英语：Deltoid curve），是三尖瓣的內擺線。
• 方圓形，n = 4，且a = b的超橢圓，看起來像是「正方形的輪子」。
  • 勒洛三角形，看起來像是「三角形的輪子」。
• 超公式（英语：Superformula），超橢圓的延伸。
• 超二次曲面（英语：Superquadrics），三維下的超橢圓。
• 超橢圓曲線（英语：Superelliptic curve），方程為Yⁿ = f(X)的曲線。

參考資料